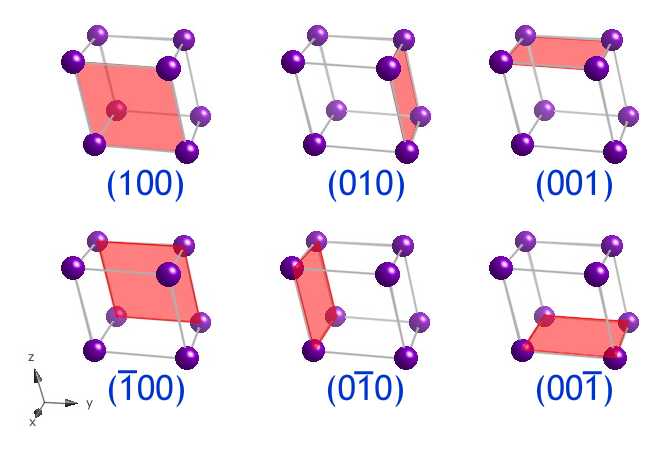
The (100), (010), (001), ( 1 00), (0 1 0) and (00 1 ) planes form the faces of the unit cell. Here, they are shown as the faces of a triclinic (a ≠ b ≠ c, α ≠ β ≠ γ ) unit cell . Although in this image, the (100) and ( 1 00) planes are shown as the front and back of the unit cell, both indices refer to the same family of planes, as explained in the animation Parallel lattice planes. It should be noted that these six planes are not all symmetrically related, as they are in the cubic system.

The (101), (110), (011), (10 1 ), (1 1 0) and (01 1 ) planes form the sections through the diagonals of the unit cell, along with those planes whose indices are the negative of these. In the image the planes are shown in a different triclinic unit cell.

The (111) type planes in a face centred cubic lattice are the close packed planes.
Click and drag on the image below to see how a close packed (111) plane intersects the fcc unit cell.
Your browser does not support the video tag.
VR rotating image
2004-2024 University of Cambridge.
Except where otherwise noted, content is licensed under a
Creative Commons Attribution - NonCommercial-ShareAlike 4.0 International.